Quasi Fermi level

A Quasi Fermi level (also called imref) is a term used in quantum mechanics and especially in solid state physics for the new Fermi level that describes the population of each type of charge carrier (electrons and holes) in a semiconductor separately when their populations are displaced from equilibrium. This displacement could be caused by the application of an external electric potential, which injects electrons and holes, or by exposure to light of energy 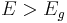$E>E_g$ , which increases the density of both electrons and holes above their equilibrium values. The displacement from equilibrium is such that the carrier populations can no longer be described by a single Fermi level, and separate quasi-Fermi levels must be used for each charge carrier.

(In this article the term "Fermi level" is used to mean the same thing as chemical potential. This is the most common usage in semiconductor physics, although in other areas of solid-state physics the term "Fermi level" means something different, namely the chemical potential at zero temperature.)

When a semiconductor is in thermal equilibrium, the distribution function of the electrons at the energy level of E is presented by Fermi-Dirac distribution function. In this case the Fermi level is defined as the level in which the probability of occupation of electron at that energy is 1/2. In thermal equilibrium, the electron quasi-Fermi level, hole quasi-Fermi level and Fermi level are equal.

When a disturbance from a thermal equilibrium situation occurs, the populations of holes and electrons change. If the disturbance is not too great or not changing too quickly, the populations of electrons and holes each relax to a state of quasi thermal equilibrium. Because the relaxation time for electrons within the conduction band is much lower than across the band gap, we can consider that the electrons are in thermal equilibrium in the conduction band. This is also applicable for holes in the valence band. In the case of electrons we can define a quasi Fermi level and temperature due to thermal equilibrium of electrons in conduction band and quasi Fermi level for holes similarly.

We can state the general Fermi function for electrons in conduction band as

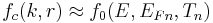
$f_c(k,r)\approx f_0(E,E_{Fn},T_n)$

and for holes in valence band as

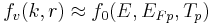
$f_v(k,r)\approx f_0(E,E_{Fp},T_p)$

where:

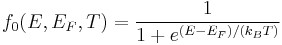$f_0(E,E_F,T)=\frac{1}{1%2Be^{(E-E_F)/(k_BT)}}$ is the Fermi-Dirac distribution function,
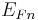$E_{Fn}$ is the electron quasi-fermi level at location r,
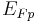$E_{Fp}$ is the hole quasi-fermi level at location r,
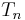$T_n$ is the electron temperature,
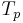$T_p$ is the hole temperature,
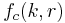$f_c(k,r)$ is the probability that a particular conduction-band state, with wavevector k and position r, is occupied by an electron,
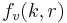$f_v(k,r)$ is the probability that a particular valence-band state, with wavevector k and position r, is occupied by an electron, i.e. not occupied by a hole,
$E$ is the energy of the conduction- or valence-band state in question,
$k_B$ is Boltzmann's constant.

This simplification will help us in many areas. For example, we can use the same equation for electron and hole densities used in thermal equilibrium, but substituting the Fermi levels and temperature. i.e.:

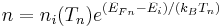
$n=n_i(T_n) e^{(E_{Fn}-E_i)/(k_BT_n)}$

and

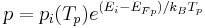
$p=p_i(T_p) e^{(E_i-E_{Fp})/k_BT_p}$

where

$n$ is the density (number per unit volume) of conduction electrons,
$p$ is the density of holes,
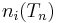$n_i(T_n)$ is the density of conduction electrons that would be present in thermal equilibrium if the Fermi level were at 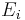$E_i$ and the temperature were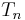 $T_n$
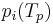$p_i(T_p)$ is the density of holes that would be present in thermal equilibrium if the Fermi level were at 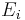$E_i$ and the temperature were $T_p$ ,
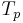This equation is valid in the Boltzmann approximation, i.e. assuming the electron and hole densities are not too high.

A current (due to the combined effects of drift and diffusion) will only flow if there is a variation in the Fermi or quasi Fermi level. The current density for electron flow can be shown to be proportional to the gradient in the electron quasi Fermi level as such:

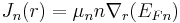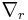$J_n(r)=\mu_nn\nabla_r(E_{Fn})$ , where $\mu$ is the electron mobility, and $\nabla_r$ is the gradient with respect to distance r.

Similarly, for holes, we have

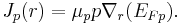
$J_p(r)=\mu_pp\nabla_r(E_{Fp}).$

Notes

Nelson, Jenny. The Physics of Solar Cells. Imperial College Press, 2003.